i could have a simple mistake in my solve. I don't have a calculator and am having to google things like sqrt 32 and round off answers. Let me get to the office Monday where I have a calculator that I am used to. I know my method to solve it. I would have to google the "two tangent theorm" to even know what it is.QillerDaemon wrote: ↑Sun Apr 02, 2023 2:38 pm Good try, but unfortunately wrong. The answer is an integer with three places, and over three times as big as your answer. While there are other ways to solve the problem, the two tangent theorem makes it easy. Problems like this, unless they involve solutions on circular diagrams, are almost always integer answers, and even if circular, almost involve integer multiples of pi or easy fractions of pi.
The two tangent theorem states that two line segments tangent to the same circle that meet at a point outside the circle have the same length from the tangent point to the outside point, ie congruent. That helps you set up formulas for the lengths of the unknown sides of the triangle. Pythagorean theorem and Bob's your uncle.
Interesting Math Problems
Moderator: Animal
- Animal
- The Great Pretender
- Posts: 29658
- Joined: Mon Jan 21, 2019 11:18 pm
Re: Interesting Math Problems
- Animal
- The Great Pretender
- Posts: 29658
- Joined: Mon Jan 21, 2019 11:18 pm
Re: Interesting Math Problems
I was trying to base my answer on a right triangle with sides of 32 and 75.69. Which would yield an area of 1211. I have no idea what I multiplied to get the answer I posted earlier. Which is still not an integer with 3 places. So I must have something wrong in the early math.
Again, let me get to my calculator.
Again, let me get to my calculator.
- stonedmegman
- In search of vitamin "T"
- Posts: 3808
- Joined: Sat Oct 02, 2021 11:41 pm
- Location: Looking for Dave
- Animal
- The Great Pretender
- Posts: 29658
- Joined: Mon Jan 21, 2019 11:18 pm
Re: Interesting Math Problems
okay. got to my desk and my calculator. My first mistake was in my quick sketch of what you said and I had something wrong.QillerDaemon wrote: ↑Sun Apr 02, 2023 2:38 pm Good try, but unfortunately wrong. The answer is an integer with three places, and over three times as big as your answer. While there are other ways to solve the problem, the two tangent theorem makes it easy. Problems like this, unless they involve solutions on circular diagrams, are almost always integer answers, and even if circular, almost involve integer multiples of pi or easy fractions of pi.
The two tangent theorem states that two line segments tangent to the same circle that meet at a point outside the circle have the same length from the tangent point to the outside point, ie congruent. That helps you set up formulas for the lengths of the unknown sides of the triangle. Pythagorean theorem and Bob's your uncle.
Anyway, after relooking at it, I'm ready to lock in my answer:
(1/2)Theta = invtan (12/20) which is 30.96 degrees. So, theta = 61.9275 degrees.
Now, the long side of the triangle would be: tanTheta * 32 or tan 61.9275 * 32 = 60.
So, the triangle is 60 x 32. Which makes the area (60)(32)(1/2) = 960.
- Bluespruce1964
- Tomorrow's Best Poster
- Posts: 4143
- Joined: Wed Jan 27, 2021 7:04 pm
- Location: Oregon
- Interests: boating, fishing, dating, travel, music, trivia, crossword puzzles, coffee, reading, photography, swimming, grilling, smoking, building
- Occupation: Interloper
Re: Interesting Math Problems
I'm up early.
- QillerDaemon
- ( ͡° ͜ʖ ͡°)
- Posts: 4423
- Joined: Sun Jan 06, 2019 9:35 pm
- Location: Beautiful downtown OrloVista FL
- Interests: キラーデモン
- Occupation: Pet the damn cat!
Re: Interesting Math Problems
The correct answer, good buddy! The hint was a suggestion to use for a geometric solution, but you used a(n equally valid) trigonometric solution. Whatever gets the answer. The two tangent theorem (TTT) is useful at odd times. Imagine a dunce cap on CTC's round idiot noggin. No matter how you place the cap on his dome, the two lengths from where the cap touches his coconut to the tip of the cap are of equal length.
Put the triangle so the longer length sits on the base and the shorter length is to the left. Draw the tangent circle to all three sides. Drop a segment from the circle's center down to the base leg, and another segment from the center to the left leg. With the triangle's right angle and these two lengths, it makes a square of 12 units on each side. More important, it shows that the left leg is made of two sub-segments of 12 units and (32 - 12 =) 20 units. It also shows that the base leg is also made up of two sub-segments of 12 units and an unknown length X. These sub-segments are divided where the circle touches each leg of the triangle. And lastly, by the TTT, the hypotenuse is made up of two sub-segments of 20 units and some unknown length. The 20 unit length passes from the left leg, around the crown of the circle, and over to the hypotenuse.
Going to the point where the hypotenuse meets the base leg, the TTT again shows that the two unknown lengths are exactly the same, X. So now we know all we need to set up the Pythagorean Theorem (PT). The left leg length is 32 (given), the base leg is (X + 12), and the hypotenuse is (X + 20). Putting these values into the PT --> 322 + (X + 12)2 = (X + 20)2, Expand, drop, and reduce, this settles down to X = 48 units. So we now know that the left leg is 32 units, the base leg is (48 + 12 =) 60 units, and we don't need to know that the hypotenuse is (48 + 20 =) 68 units.
From the formula for the area of a triangle, A = 1/2(B)(H), B = 60 units, H = 32 units, and solving, A = 960 units2.
Put the triangle so the longer length sits on the base and the shorter length is to the left. Draw the tangent circle to all three sides. Drop a segment from the circle's center down to the base leg, and another segment from the center to the left leg. With the triangle's right angle and these two lengths, it makes a square of 12 units on each side. More important, it shows that the left leg is made of two sub-segments of 12 units and (32 - 12 =) 20 units. It also shows that the base leg is also made up of two sub-segments of 12 units and an unknown length X. These sub-segments are divided where the circle touches each leg of the triangle. And lastly, by the TTT, the hypotenuse is made up of two sub-segments of 20 units and some unknown length. The 20 unit length passes from the left leg, around the crown of the circle, and over to the hypotenuse.
Going to the point where the hypotenuse meets the base leg, the TTT again shows that the two unknown lengths are exactly the same, X. So now we know all we need to set up the Pythagorean Theorem (PT). The left leg length is 32 (given), the base leg is (X + 12), and the hypotenuse is (X + 20). Putting these values into the PT --> 322 + (X + 12)2 = (X + 20)2, Expand, drop, and reduce, this settles down to X = 48 units. So we now know that the left leg is 32 units, the base leg is (48 + 12 =) 60 units, and we don't need to know that the hypotenuse is (48 + 20 =) 68 units.
From the formula for the area of a triangle, A = 1/2(B)(H), B = 60 units, H = 32 units, and solving, A = 960 units2.
If you can't be a good example, you can still serve as a horrible warning.
“All mushrooms are edible. Some even more than once!”
これを グーグル 翻訳に登録してくれておめでとう、バカ。
“All mushrooms are edible. Some even more than once!”
これを グーグル 翻訳に登録してくれておめでとう、バカ。
- Reservoir Dog
- Ricky
- Posts: 14475
- Joined: Mon Jan 07, 2019 4:32 pm
- Location: Kicking and a' gouging in the mud and the blood and the beer.
- Animal
- The Great Pretender
- Posts: 29658
- Joined: Mon Jan 21, 2019 11:18 pm
Re: Interesting Math Problems
Honestly, I don't ever remember that formula,QillerDaemon wrote: ↑Wed Apr 05, 2023 5:51 pm The correct answer, good buddy! The hint was a suggestion to use for a geometric solution, but you used a(n equally valid) trigonometric solution. Whatever gets the answer. The two tangent theorem (TTT) is useful at odd times. Imagine a dunce cap on CTC's round idiot noggin. No matter how you place the cap on his dome, the two lengths from where the cap touches his coconut to the tip of the cap are of equal length.
Put the triangle so the longer length sits on the base and the shorter length is to the left. Draw the tangent circle to all three sides. Drop a segment from the circle's center down to the base leg, and another segment from the center to the left leg. With the triangle's right angle and these two lengths, it makes a square of 12 units on each side. More important, it shows that the left leg is made of two sub-segments of 12 units and (32 - 12 =) 20 units. It also shows that the base leg is also made up of two sub-segments of 12 units and an unknown length X. These sub-segments are divided where the circle touches each leg of the triangle. And lastly, by the TTT, the hypotenuse is made up of two sub-segments of 20 units and some unknown length. The 20 unit length passes from the left leg, around the crown of the circle, and over to the hypotenuse.
Going to the point where the hypotenuse meets the base leg, the TTT again shows that the two unknown lengths are exactly the same, X. So now we know all we need to set up the Pythagorean Theorem (PT). The left leg length is 32 (given), the base leg is (X + 12), and the hypotenuse is (X + 20). Putting these values into the PT --> 322 + (X + 12)2 = (X + 20)2, Expand, drop, and reduce, this settles down to X = 48 units. So we now know that the left leg is 32 units, the base leg is (48 + 12 =) 60 units, and we don't need to know that the hypotenuse is (48 + 20 =) 68 units.
From the formula for the area of a triangle, A = 1/2(B)(H), B = 60 units, H = 32 units, and solving, A = 960 units2.
This problem did bring up an interesting thought, though. Obviously this triangle is unique in that all three sides are integers. 32, 60 and 60. The most famous of those triangles is the 3,4, 5.
So, is there a formula to determine the size of a circle that is tangent to all three sides of a right triangle?
- QillerDaemon
- ( ͡° ͜ʖ ͡°)
- Posts: 4423
- Joined: Sun Jan 06, 2019 9:35 pm
- Location: Beautiful downtown OrloVista FL
- Interests: キラーデモン
- Occupation: Pet the damn cat!
Re: Interesting Math Problems
Yea, it's one of those tools you forget about, stuck in the bottom of your tool chest, with all sorts of more usable tools piled on top of it. And then, you come across a problem and that's just the tool to fix it. Since you used trig, you didn't need it.Animal wrote: ↑Wed Apr 05, 2023 6:45 pm
Honestly, I don't ever remember that formula,
This problem did bring up an interesting thought, though. Obviously this triangle is unique in that all three sides are integers. 32, 60 and 60. The most famous of those triangles is the 3,4, 5.
So, is there a formula to determine the size of a circle that is tangent to all three sides of a right triangle?
Those are Pythagorean triplets. The 3-4-5 is the simplest one, so is 5-12-13. And the triangle in the original problem is also a triple when reduced down to lowest terms -> 8-15-17 (32/4, 60/4, 68/4). It'd be an interesting question whether any triangle made up of a Pythagorean triple has an inscribed circle whose radius is also an integer.
And yes, there is such a formula for that. The radius of an inscribed circle tangent to all sides is (1/2)[(side A) + (side B) - (hypotenuse)]. That's based on Heron's formula, and I had to Google all that. For the triangle in the original problem, (1/2)[32 + 60 - 68] = (1/2)[24] = 12.
So for 3-4-5, the inscribed radius is 1, for 5-12-13, it's 2, and for 7-24-25, it's 3. Also notice with all of those triples, the base and the hypotenuse are often so close together in value, it kind of reminds of how sin(θ) ≈ θ (in radians) for values of θ < 14°.
If you can't be a good example, you can still serve as a horrible warning.
“All mushrooms are edible. Some even more than once!”
これを グーグル 翻訳に登録してくれておめでとう、バカ。
“All mushrooms are edible. Some even more than once!”
これを グーグル 翻訳に登録してくれておめでとう、バカ。
-
disco.moon
- Nicely requested a nicer title.
- Posts: 3215
- Joined: Tue Nov 17, 2020 8:11 pm
Re: Interesting Math Problems
I like to check in this thread to remind myself how stupid I am. ...
.
.
.
Ahhhh that'll do it.

.
.
.
Ahhhh that'll do it.

- Animal
- The Great Pretender
- Posts: 29658
- Joined: Mon Jan 21, 2019 11:18 pm
Re: Interesting Math Problems
So, if a triangle has integer sides (3-4-5 or 5-12-13) then the inscribed circle has a radius that is an integer? Is that true in every case?QillerDaemon wrote: ↑Thu Apr 06, 2023 3:11 amYea, it's one of those tools you forget about, stuck in the bottom of your tool chest, with all sorts of more usable tools piled on top of it. And then, you come across a problem and that's just the tool to fix it. Since you used trig, you didn't need it.Animal wrote: ↑Wed Apr 05, 2023 6:45 pm
Honestly, I don't ever remember that formula,
This problem did bring up an interesting thought, though. Obviously this triangle is unique in that all three sides are integers. 32, 60 and 60. The most famous of those triangles is the 3,4, 5.
So, is there a formula to determine the size of a circle that is tangent to all three sides of a right triangle?
Those are Pythagorean triplets. The 3-4-5 is the simplest one, so is 5-12-13. And the triangle in the original problem is also a triple when reduced down to lowest terms -> 8-15-17 (32/4, 60/4, 68/4). It'd be an interesting question whether any triangle made up of a Pythagorean triple has an inscribed circle whose radius is also an integer.
And yes, there is such a formula for that. The radius of an inscribed circle tangent to all sides is (1/2)[(side A) + (side B) - (hypotenuse)]. That's based on Heron's formula, and I had to Google all that. For the triangle in the original problem, (1/2)[32 + 60 - 68] = (1/2)[24] = 12.
So for 3-4-5, the inscribed radius is 1, for 5-12-13, it's 2, and for 7-24-25, it's 3. Also notice with all of those triples, the base and the hypotenuse are often so close together in value, it kind of reminds of how sin(θ) ≈ θ (in radians) for values of θ < 14°.
- Animal
- The Great Pretender
- Posts: 29658
- Joined: Mon Jan 21, 2019 11:18 pm
Re: Interesting Math Problems
This formula made me wonder. In your original problem, you knew that one side was 32 (given in the question). And you knew the radius of the "inscribed circle" to be 12.QillerDaemon wrote: ↑Thu Apr 06, 2023 3:11 amThe radius of an inscribed circle tangent to all sides is (1/2)[(side A) + (side B) - (hypotenuse)].
If you had the formula above, could you solve for Side B and the hypotenuse? I think you could solve the original problem with that formula.
Substitute the radius for 12, side A for 32, and the hypotenuse for (32^2 + B^2)^(1/2)
12 = (1/2)[(32) + B - (32^2) + B^2)^(1/2)] and solve for B. Then get the area.
- saltydog
- Chief Biden Ballwasher
- Posts: 5112
- Joined: Tue Oct 26, 2021 3:36 pm
- Location: Western East Coast
Re: Interesting Math Problems
Math nerds are a completely different breed of human. My cousin teaches high level math graduate courses and some of the stuff he randomly posts on social media is almost NASA-level aptitude.disco.moon wrote: ↑Thu Apr 06, 2023 10:08 am I like to check in this thread to remind myself how stupid I am. ...
.
.
.
Ahhhh that'll do it.

The problem is not that there is evil in the world, the problem is that there is good. Because otherwise, who would care?
- QillerDaemon
- ( ͡° ͜ʖ ͡°)
- Posts: 4423
- Joined: Sun Jan 06, 2019 9:35 pm
- Location: Beautiful downtown OrloVista FL
- Interests: キラーデモン
- Occupation: Pet the damn cat!
Re: Interesting Math Problems
I tried about twenty triples, and they all produced an integer radius. But that's not a proof. And mathematical proofs were never a strong point for me. Only takes one negative case to disprove the whole notion, but maybe a proof by contradiction. Like D.M, I'm just not that smart...
Another formula for finding the radius of an inscribed triangle, not necessarily a right triangle, also comes ultimately from Heron's formula: if A is the area of the triangle, and P is its perimeter, then the radius of the inscribed circle is R=2A/P. In fact, all those formulas come from Heron's formula, just rearranged in various ways for various goals. So I think what you asked about in your second reply is true, just working backwards.
If you can't be a good example, you can still serve as a horrible warning.
“All mushrooms are edible. Some even more than once!”
これを グーグル 翻訳に登録してくれておめでとう、バカ。
“All mushrooms are edible. Some even more than once!”
これを グーグル 翻訳に登録してくれておめでとう、バカ。
- Animal
- The Great Pretender
- Posts: 29658
- Joined: Mon Jan 21, 2019 11:18 pm
Re: Interesting Math Problems
damn, that's a simple formula. R=2A/P.QillerDaemon wrote: ↑Thu Apr 06, 2023 3:56 pmI tried about twenty triples, and they all produced an integer radius. But that's not a proof. And mathematical proofs were never a strong point for me. Only takes one negative case to disprove the whole notion, but maybe a proof by contradiction. Like D.M, I'm just not that smart...
Another formula for finding the radius of an inscribed triangle, not necessarily a right triangle, also comes ultimately from Heron's formula: if A is the area of the triangle, and P is its perimeter, then the radius of the inscribed circle is R=2A/P. In fact, all those formulas come from Heron's formula, just rearranged in various ways for various goals. So I think what you asked about in your second reply is true, just working backwards.
For your example, the sides were 32, 60, 68. R=2(960)/160 = 12. So that works.
I don't know why that is kind of mind blowing to me, that all of these integer triangles also have integer radiuses on the inscribed triangles. yet, i don't see a very simple proof to it.
- QillerDaemon
- ( ͡° ͜ʖ ͡°)
- Posts: 4423
- Joined: Sun Jan 06, 2019 9:35 pm
- Location: Beautiful downtown OrloVista FL
- Interests: キラーデモン
- Occupation: Pet the damn cat!
Re: Interesting Math Problems
Nah, not at all. As Animal mentioned, math nerds are their own species. Back in the mid 80's I wanted to go into computer science. But at the time, and probably still true, they did not allow major transfers, and I was an English major. Either you started out in the CS dept as a freshman, or you didn't and had to find another major. But the Math dept had a "back door" CS degree: BS in Math with an automatic minor in CS. Basically a CS degree heavy in math. I'd have to take a base set of math classes, but would be allowed take practically any CS class I wanted. That lasted about a year, not due to the CS classes but due to the upper level math classes. At that level, it's almost never about formulas and finding answers, it's all about mathematical proofs to various propositions. Logic and lots of it! I hung around a few math nerds, and it was very discouraging, they were like in a different thought universe. My mind didn't work like that, and I simply couldn't make it. Got all A's in the CS classes, and almost failed at the math classes.disco.moon wrote: ↑Thu Apr 06, 2023 10:08 am I like to check in this thread to remind myself how stupid I am. ...
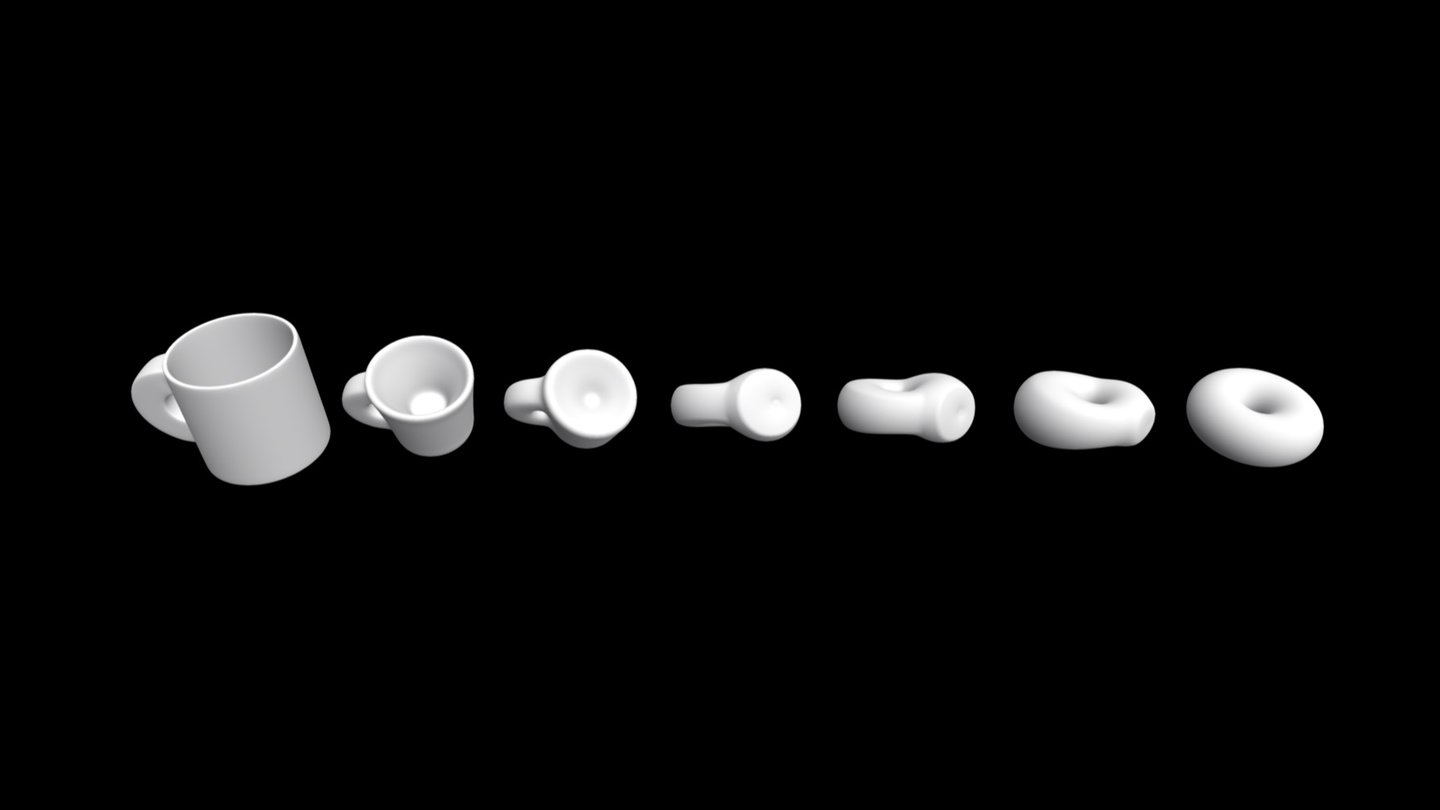
Take topology, the math of shapes and their properties and relationships. That's the math that takes a donut and reshapes it into a coffee cup. Pretty esoteric, huh? In a typical topology text, that covered on page 2. The rest of the 475 pages? Might as well be reading a wizard's spell book.
After a year, I changed over to biochemistry with a chemistry minor, and never looked back, except when it came to physical chemistry, which I did rather poorly in. But biochemistry was a real blast, it was just at the cusp of "quick" DNA testing (which still took weeks and a large clean specimen), and we couldn't help but wonder where it was leading to. My own interest was biochemical evolution, how the chemistry of life formed. Really interesting stuff!
If you can't be a good example, you can still serve as a horrible warning.
“All mushrooms are edible. Some even more than once!”
これを グーグル 翻訳に登録してくれておめでとう、バカ。
“All mushrooms are edible. Some even more than once!”
これを グーグル 翻訳に登録してくれておめでとう、バカ。
- Animal
- The Great Pretender
- Posts: 29658
- Joined: Mon Jan 21, 2019 11:18 pm
Re: Interesting Math Problems
If you start with the Radius that you want an inscribed triangle (r). And you have a right triangle with sides a, b, c. Then you can create it for any integer value of r like this:
r = radius (any integer).
a = 2r +1
b = 2r(r+1)
c = 2r(r+1) + 1
and all of them will be integers. and the triangle will be a right triangle.
r = radius (any integer).
a = 2r +1
b = 2r(r+1)
c = 2r(r+1) + 1
and all of them will be integers. and the triangle will be a right triangle.
-
disco.moon
- Nicely requested a nicer title.
- Posts: 3215
- Joined: Tue Nov 17, 2020 8:11 pm
Re: Interesting Math Problems
That sounds cool. My lack of math knowledge wasn't on the radar of my mother. Its really cool that you guys know this stuff I'm envious.QillerDaemon wrote: ↑Thu Apr 06, 2023 4:19 pmNah, not at all. As Animal mentioned, math nerds are their own species. Back in the mid 80's I wanted to go into computer science. But at the time, and probably still true, they did not allow major transfers, and I was an English major. Either you started out in the CS dept as a freshman, or you didn't and had to find another major. But the Math dept had a "back door" CS degree: BS in Math with an automatic minor in CS. Basically a CS degree heavy in math. I'd have to take a base set of math classes, but would be allowed take practically any CS class I wanted. That lasted about a year, not due to the CS classes but due to the upper level math classes. At that level, it's almost never about formulas and finding answers, it's all about mathematical proofs to various propositions. Logic and lots of it! I hung around a few math nerds, and it was very discouraging, they were like in a different thought universe. My mind didn't work like that, and I simply couldn't make it. Got all A's in the CS classes, and almost failed at the math classes.disco.moon wrote: ↑Thu Apr 06, 2023 10:08 am I like to check in this thread to remind myself how stupid I am. ...
Take topology, the math of shapes and their properties and relationships. That's the math that takes a donut and reshapes it into a coffee cup. Pretty esoteric, huh? In a typical topology text, that covered on page 2. The rest of the 475 pages? Might as well be reading a wizard's spell book.
After a year, I changed over to biochemistry with a chemistry minor, and never looked back, except when it came to physical chemistry, which I did rather poorly in. But biochemistry was a real blast, it was just at the cusp of "quick" DNA testing (which still took weeks and a large clean specimen), and we couldn't help but wonder where it was leading to. My own interest was biochemical evolution, how the chemistry of life formed. Really interesting stuff!
- QillerDaemon
- ( ͡° ͜ʖ ͡°)
- Posts: 4423
- Joined: Sun Jan 06, 2019 9:35 pm
- Location: Beautiful downtown OrloVista FL
- Interests: キラーデモン
- Occupation: Pet the damn cat!
Re: Interesting Math Problems

If you can't be a good example, you can still serve as a horrible warning.
“All mushrooms are edible. Some even more than once!”
これを グーグル 翻訳に登録してくれておめでとう、バカ。
“All mushrooms are edible. Some even more than once!”
これを グーグル 翻訳に登録してくれておめでとう、バカ。
-
Antknot
- Not Nearly As Old As Who
- Posts: 7692
- Joined: Sun Jan 06, 2019 11:30 pm
- Location: South Carolina
Re: Interesting Math Problems
Think.about it, you’re a donut.
- QillerDaemon
- ( ͡° ͜ʖ ͡°)
- Posts: 4423
- Joined: Sun Jan 06, 2019 9:35 pm
- Location: Beautiful downtown OrloVista FL
- Interests: キラーデモン
- Occupation: Pet the damn cat!
Re: Interesting Math Problems
I also thought the same damn thing: the radius of the inscribed tangent circle is calculated by two values that don't on first look to have anything with the circle. The formula might as well calculate something about an inscribed square or some shape that the triangle fits into. But the formula relates the radius with the area and perimeter of the triangle, qed. If you go thru a proof of it from Heron's formula, the circle bits basically drop out, leaving the area and perimeter. All you can do is go "well, golly!..." Math can be so magical, even if the words look like wizards' spells. Which they probably are...Animal wrote: ↑Thu Apr 06, 2023 4:01 pm damn, that's a simple formula. R=2A/P.
For your example, the sides were 32, 60, 68. R=2(960)/160 = 12. So that works.
I don't know why that is kind of mind blowing to me, that all of these integer triangles also have integer radiuses on the inscribed triangles. yet, i don't see a very simple proof to it.
As for the integer values, I think I might ask one of the Youtube math geeks their opinion on it.
If you can't be a good example, you can still serve as a horrible warning.
“All mushrooms are edible. Some even more than once!”
これを グーグル 翻訳に登録してくれておめでとう、バカ。
“All mushrooms are edible. Some even more than once!”
これを グーグル 翻訳に登録してくれておめでとう、バカ。
- stonedmegman
- In search of vitamin "T"
- Posts: 3808
- Joined: Sat Oct 02, 2021 11:41 pm
- Location: Looking for Dave
Re: Interesting Math Problems
QillerDaemon wrote: ↑Wed Apr 05, 2023 5:51 pm Imagine a dunce cap on CTC's round idiot noggin. No matter how you place the cap on his dome, the two lengths from where the cap touches his coconut to the tip of the cap are of equal.
Be careful when you follow the masses. Sometimes the "M" is silent
-
rule34
- No life apart from this stupid forum board
- Posts: 1646
- Joined: Tue Jan 19, 2021 7:08 pm
Re: Interesting Math Problems
If you lack $14.00 having 47 cents how much money do you have?
du.du.du.dude looks like a lady
- Reservoir Dog
- Ricky
- Posts: 14475
- Joined: Mon Jan 07, 2019 4:32 pm
- Location: Kicking and a' gouging in the mud and the blood and the beer.
- QillerDaemon
- ( ͡° ͜ʖ ͡°)
- Posts: 4423
- Joined: Sun Jan 06, 2019 9:35 pm
- Location: Beautiful downtown OrloVista FL
- Interests: キラーデモン
- Occupation: Pet the damn cat!
Re: Interesting Math Problems
Two circles of radius 7 units, A and B, are tangent to one another. Another circle of radius 7 units, O, overlaps circles A and B. The diameter of circle O sits on the same line that is tangent to the tops of circles A and B. What is the area of the overlap of circle O over circles A and B?
If you can't be a good example, you can still serve as a horrible warning.
“All mushrooms are edible. Some even more than once!”
これを グーグル 翻訳に登録してくれておめでとう、バカ。
“All mushrooms are edible. Some even more than once!”
これを グーグル 翻訳に登録してくれておめでとう、バカ。

